√完了しました！ if f(x)=x-1/x 1 then f(2x) is equal to 102050

Now, if we plug in 2x to this we get g (2x) = 4x^22x Clearly that's not right, so we need to adjust To make f (x) equal g (x), we must need to change each individual term to be the same To this most simply, multiply the x^2 term by 1/4 and the x term by 1/2 This will give us g (x) = 1/4 (x^2)1/2 (x) Let's checkAns f(x)=x/(x1) f(x1)=(x1)/x Hence f(a)/f(a1)=a²/(a² 1) =f(a²) (Ans) Please feel free to post as many doubts on our discussion forum as you can If you find any question Difficult to understand post it here and we will get you the answer and detailed solution very quickly WeSOLUTION If f(x)= x2/x1, then f(n1) is equal to A)1/2 b)n2/n1 c)n1/n2 d)n1/n2 Algebra > Rationalfunctions> SOLUTION If f(x)= x2/x1, then f(n1) is equal to A)1/2 b)n2/n1 c)n1/n2 d)n1/n2 Log On Algebra Rational Functions, analyzing and

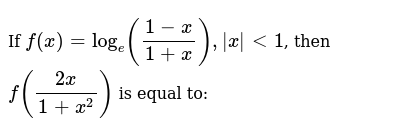
If F X Log E 1 X 1 X X Lt1 Then F 2x 1 X 2 Is

If f(x)=x-1/x 1 then f(2x) is equal to

If f(x)=x-1/x 1 then f(2x) is equal to-• (a) The kth derivative of ex is ex, which is equal to 1 at x = 0, so the kth Taylor coefficient of f(x) = ex at zero is ak = f(k)(0) k!If a horizontal line intersects the graph of f(x) in more than one point, then f(x) is not onetoone The reason f(x) would not be onetoone is that the graph would contain two points that have the same second coordinate – for example, (2,3) and (4,3) That would mean that f(2) and f(4) both equal 3, and onetoone

If F R R Is Defined By F X 2x 3 How Do You Prove That F Is A Bijection And Find Its Inverse Quora

Answer to For the function defined by f(x)={x^2, x less than equal to 1 2x1, x greater than 1} Define f(0) and also draw the graph for f(x)?If 2f(x) 3f(1/x) = x2 (x ≠ 0) then f(2) is equal to A \(\frac{7}{4}\) B \(\frac{5}{2}\) C 1 D None of these Two forces having magnitudes 3F and 2F, when act in the same direction simultaneously on a body, the net force is equal to 25 N Find the value of F If f (x) = 2x 1 and g(x) = (x − 1) / 2 for all real x, then (fog)^ (–1) (1/x) is equal to asked in Sets, Relations and Functions by Maahi01 (

If 2f (X) − 3 F ( 1 X ) = X 2 (X ≠ 0), Then F(2) is Equal to (A) − 7 4 (B) 5 2 −1 (D) None of These Department of PreUniversity Education, Karnataka PUC Karnataka Science Class 11 Textbook Solutions 71 Important Solutions 3 Question Bank Solutions 51 Concept NotesIf F ( X ) = Log ( 1 X 1 − X ) , Then F ( 2 X 1 X 2 ) is Equal To(A) {F(X)}2 (B) {F(X)}3 2f(X) (D) 3f(X) Department of PreUniversity Education, Karnataka PUC Karnataka Science Class 11 Textbook Solutions 71 Important Solutions 3 Question Bank Solutions 51 Concept Notes & Videos 531 Syllabus Advertisement If the derivative of \(\dfrac{e^{3x} e^x}{e^{4x} e^{2x} 1}\) if f(ex ex) C, then f(x) is equal to Q7 Let f be a differentiable function defined for all x ∈ R such that f(x3) = x5 for all x ∈ R, x ≠ 0

Here's the graph Then the inverse is y = (–2x – 2) / (x – 1), and the inverse is also a function, with domain of all x not equal to 1 and range of all y not equal to –2 Find the inverse of f ( x) = x2 – 3 x 2, x < 15 With the domain restriction, the graph looks like thisIf f (x) = 3/x 2 and g (x) = 1/x, then f compositefunction g)(x) is equal to (a) 3x/1 2x (b) 3x/x 2 (c) 3/x 2 (d) 1 2x/3 x (e) none of these If (3, 5) is a point on the graph of y = f(x), what are the coordinates of the corresponding point on the graph of y = 2f (x 3)? the blue line is the graph of #f(2x1)#, so here, the equation of #f(2x 1) = 2x 1# this is only true when #f(x) = x 2# , which is the graph shown in red Answer link

Verifying Inverse Functions By Composition Not Inverse Video Khan Academy

If F X 2x 8 Then F 1 1 Is Equal To Brainly In

David Joyce , Professor Emeritus of Mathematics at Clark University Author has 45K answers and 44M answer views Given F (2x1) = x2 Put 2x1 = y=> x = (y1)/2 Hence F (y) = (y1)/2 2 = (y5)/2 So F (x) = (x5)/2 So F (x3) = (x35)/2 = (x8)/2 65K views যদি `f(x)=(x1)/(x1)` তারপর `f(2x)` সমান Prove that the area of an equilateral triangle is equal to where is the side of the triangle In Figure, is a trapezium in which isIf f (x) = x1/x1 then f (2x) is ?

Example 10 If F 1 X X 1 Then Find F 2 H Substitute X 1 H In Given Function And Get The Value Of F 2 H Sol We Have F 1 X

How To Find F X 1 If F X 3x 5x 9 Quora

If f(x) = x 2, where x denotes the greatest integer less than or equal to x, then f'(25) is equal to If f(x) = 2 2x – 1 and ∅(x) = – 2 x 2x log 2 If f '(x) > ∅' (x), then If f(x) = log((1x)/(1x)) then f(2x/1x 2) is equal to If f(x) = sin 2 x/(1 cot x) cos 2 x/(1tan x), then f'(π/4) is equal toIf f (x) = x1/x1 then find the value of f (2x) Find the answer to this question along with unlimited Maths questions and prepare better for JEE examinationIff( x 1 2x − 1) = 2x, X ∈ N, then the value of is equal to f(2) is equal to 2 If A\B = {a, b}, B\A = {c, d} and A\capB = {e, f}, then the set B is equal to 3 The function f A →B is given by f(x) = x, xϵA, is one to one but not onto Then

If F X 2x And G X X 2 2 1 Then Which Of The Following Can Be Discontinuous Function

Let F R Gt R Be A Positive Increasing Function With Lim X Gtoo F 3x F X 1 Then Lim Youtube

Summary "Function Composition" is applying one function to the results of another (g º f) (x) = g (f (x)), first apply f (), then apply g () We must also respect the domain of the first function Some functions can be decomposed into two (or more) simpler functionsIf you mean f(x)=(2x1)/(x2), then that could be written f (x) = 2 x − 1 x 2 and then you would not need parentheses because the horizontal line functions as a grouping symbol So, to answer your question if you mean f (x) = 2 x − 1 x 2, you just plug in x=1 and get f (1) = 2 × 1 − 1 1 2 = 1• (b) The expression for the Lagrange remainder is Rn(x) = 1 (n1)!

If F X X 1 X 1 Find F 2x In Terms Of F X

If Math F 2x 1 X Math What Is Math F 2 Math Quora

Xk = 1x 1 2!Click here👆to get an answer to your question ️ If g(x) = x^2 x 1 and (gof)(x) = 4x^2 10x 5 , then f (54) is equal toIf $f \mathbb{R} \to \mathbb{R}$ is a continuous function and satisfies $f(x)=f(2x1)$, then its not to hard to show that $f$ is a constant My question is suppose

If X 1 2x 2 What Is The Value Of 8x 3 1 X 3 Quora

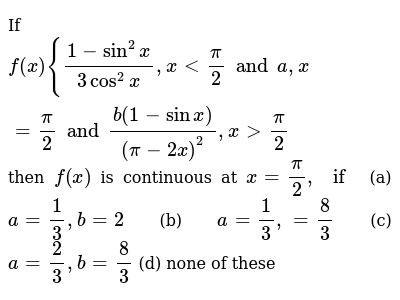
If F X 1 Sin 2x 3cos 2x Xltpi 2 And A X Pi 2 And B 1

 To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW If `f(x)=(x1)/(x1)` then `f(2x)` is equal toX2 ··· 1 n!{x 2 x ≥ 0 } "x squared such that x is greater than or equal to zero" {√x x ≥ 0 } "square root of x such that x is greater than or equal to zero" And you can see they are "mirror images" of each other about the diagonal y=x Note when we restrict the domain to x ≤ 0 (less than or equal to 0) the inverse is then f1 (x) = −√x

If F X 2x 3 3x 2 1 Then Number Of Distinct Real Solution S Of The Equation F F X 0 Is Are K Then 7k 10 2 Is Equal To

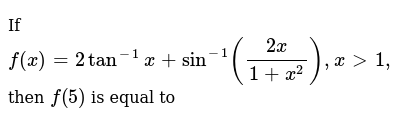
If F X 1 2x 2 What Is F 14

 If f(x) = 2x, then f 1(x) =2x ½x x 2 Answers 2 Get Other questions on the subject Mathematics Mathematics, 1500, gm2 Select the correct product ( y z ) 3 y3 z3 y3 3 y2z 3 yz2 z3 3 y 3 z y3z3 Answers 1 The functions f(x) = 2x²2x1 and g(x)=x²5x3 are equal to each others then find the value of x The estimating factor that considers the prevailing belief in some firms that detailed estimating takes too much time and is Continuity and Differentiability Class 12 MCQs Questions with Answers Question 1 If f (x) = 2x and g (x) = \(\frac{x^2}{2}\) 1, then'which of the following can be a

If F 1 X 2 X Is Given By F X X 1 X Then F 1 X Equals A X Sqrt X 2 4 2 B X 1 X 2 C X Sqrt X 2 4 2 D 1 Sqrt X 2 4

If F X X 1 X 1 Then Show That F 2x 3f X 1 F X 3 Maths Relations And Functions Meritnation Com

Let 3 f (x) − 2 f (x 1 ) = x, then f ′ (2) is equal to A 2 / 7 B 1 / 2 C 2 D 7 / 2 Hard Answer Correct option is B 1 / 2 Differentiate 3 f (x) Let f (2 x yF ^ { 1 } ( x ) = \frac { x 1 } { x 2 } f − 1 ( x) = x − 2 − x − 1 Multiply both sides of the equation by x2 Multiply both sides of the equation by x − 2 f^ {1}x\left (x2\right)=x1 f − 1 x ( x − 2) = − x − 1 Use the distributive property to multiply f^ {1}x by x2Question 5 2 pts If f(x)=x^2x1, then f(x)f(x1) is equal to ?

If F X 2x 1 X 5 e 5 Then F 1 X Is Equal To

If F X X 1 Find F 2 From The First Principle Brainly In

Answer to Find an expression for the area under the graph of f(x) = 2x/(x^2 1), 1 less than or equal to x less than or equal to 3 as a limit Do Begin by graphing f ( x) = 2 x Then use transformations of this graph to graph the given function Be sure to graph and give equations of the asymptotes Use the graphs to determine each function's domain and range If applicable, use a graphing utility to confirm your handdrawn graphs h ( x) = 2 x 1 − 1 Your answer If `f(x)=(1, x, x1), (2x, x(x1), (x1)x), (3x(x1), x(x1)(x2), (x1)x(x1))` then `f(5000)` is equal to `0` b `1` c `500` d `500` CBSE board exam 21 Class 12 result released today, Candidates who appeared for the exam can check their results now

If F X 2 X 2 5 1 Find F 1 X F 1 X 5 Chegg Com

Www3 Nd Edu Apilking Math Work Old exams Exam1f08soluutions Pdf

It must be in terms of f (x)∵ ƒ(x) = ( x 1 ) / ( x 1 ) (1) ∴ ( x 1 ) ƒ(x) = x 1 ∴ x ƒ(x) ƒ(x) = x Thank you for registering One of our academic counsellors will contact you within 1 working dayF(n1)(ξ)xn1 = 1 (n1)!Divide \frac {f1} {f}, the coefficient of the x term, by 2 to get \frac {1} {2}\frac {1} {2f} Then add the square of \frac {1} {2}\frac {1} {2f} to both sides of the equation This step makes the left hand side of the equation a perfect square Square \frac {1} {2}\frac {1} {2f}

X 1 X 1 Then Can You Prove That X 3 1 0 Quora

If F X 2x 1 3x 2 Then Fof 2 Is Equal To

The notation f −1 is sometimes also used for the inverse function of the function f, which is not in general equal to the multiplicative inverse For example, the multiplicative inverse 1/(sin x) = (sin x) −1 is the cosecant of x, and not the inverse sine of x denoted by sin −1 x or arcsin x Only for linear maps are they strongly relatedGraph f(x)=x^22x1 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for Set equal to the new right side Use the vertex form, , to determine the values of , , and Since the value of is positive, the parabola opens up Opens UpSOLUTION Using remainder theorem the given expression should be equal to zero when we put x 1 = 0, Or x = 1 ⇒ x 3 2x 2 – 5x k = 0 when x = 1 ⇒ 1 3 2 (1) 2 – 5 (1) k = 0 ⇒ k = 6 ∴ the value of k is 6

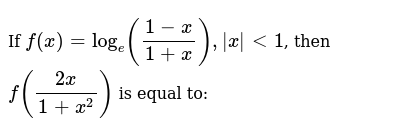
If F X Log E 1 X 1 X X Lt1 Then F 2x 1 X 2 Is

If F X X 1 X 1 Then F 2x Is Equal To

If f ( tan x) = cos 2 x, x ≠ ( 2 n 1) π 2, n ∈ I then incorrect statement is IfThe domain of the function 2 If f(x) = 1 x2 4x 4 − 4 x4 4x3 4x2 4 x3 2x2 , then f(1 2) is equal to 3 The domain of the function 4 Iff( x 1 2x − 1) = 2x, X ∈ N, then the value of is equal to f(2) is equal to 5 If n(A) = 5 and n(B) = 7, then the number of relations on A × B isIf f(x) = x 2 and g(x) = x – 1 then gf(x) = g(x 2) = x 2 – 1 fg(x) = f(x – 1) = (x – 1) 2 As you can see, fg does not necessarily equal gf The Inverse of a Function The inverse of a function is the function which reverses the effect of the original function For example the inverse of y = 2x is y = ½ x

If F X Log 1 X 1 X Then F 2x 1 X 2 Is Equal To

Misc 2 If F X X2 Find F 1 1 F 1 1 1 1 Chapter 2

About four X So here one plus four X Plus 4X to the power to upon Factorial too clothes and so on Upon Okay so now here we simplify this and here we get half of acts equal 1 1 equal to zero two X Negative four X Equal to negative to a blood 2X to the power to upon factorial tooO 2x ОО 03 03x Next > Get more help from CheggQuestion If f(x) = 2x 1 and g(x) = (x – 1)/2 , then f(g(x)) = So, I know what to do Basic plug and chug I also know that the answer is X, I just don't know how to get to the answer I plug in g(x

If A Not Equal To B And 1 X 1 A 1 B Then X Chegg Com

If F X 1 3x 4 Then What Is F X And F 4 Quora

= 1 k!, and Pn(x) = ∑n k=0 1 k!Free functions calculator explore function domain, range, intercepts, extreme points and asymptotes stepbystepE˘ xn1 for some ξ strictly between 0

Finding Decreasing Interval Given The Function Video Khan Academy

If F X Log 1 X 1 X Then F 2x 1 X 2 Is Equal To A F X 2 B F X 3 C 2f X Youtube

Graph f (x)= (2x1)/ (x1) f (x) = 2x − 1 x − 1 f ( x) = 2 x 1 x 1 Find where the expression 2x−1 x −1 2 x 1 x 1 is undefined x = 1 x = 1 Consider the rational function R(x) = axn bxm R ( x) = a x n b x m where n n is the degree of the numerator and m m is the degree of the denominator 1We start from x2f(x) f(1 − x) = 2x − x4 Replace x by 1 − x Then 1 − x gets replaced by x So (1 − x)2f(1 − x) f(x) = 2(1 − x) − (1 − x)4 Two linear equations in two unknowns, f(x) and f(1 − x) Solve for f(x)

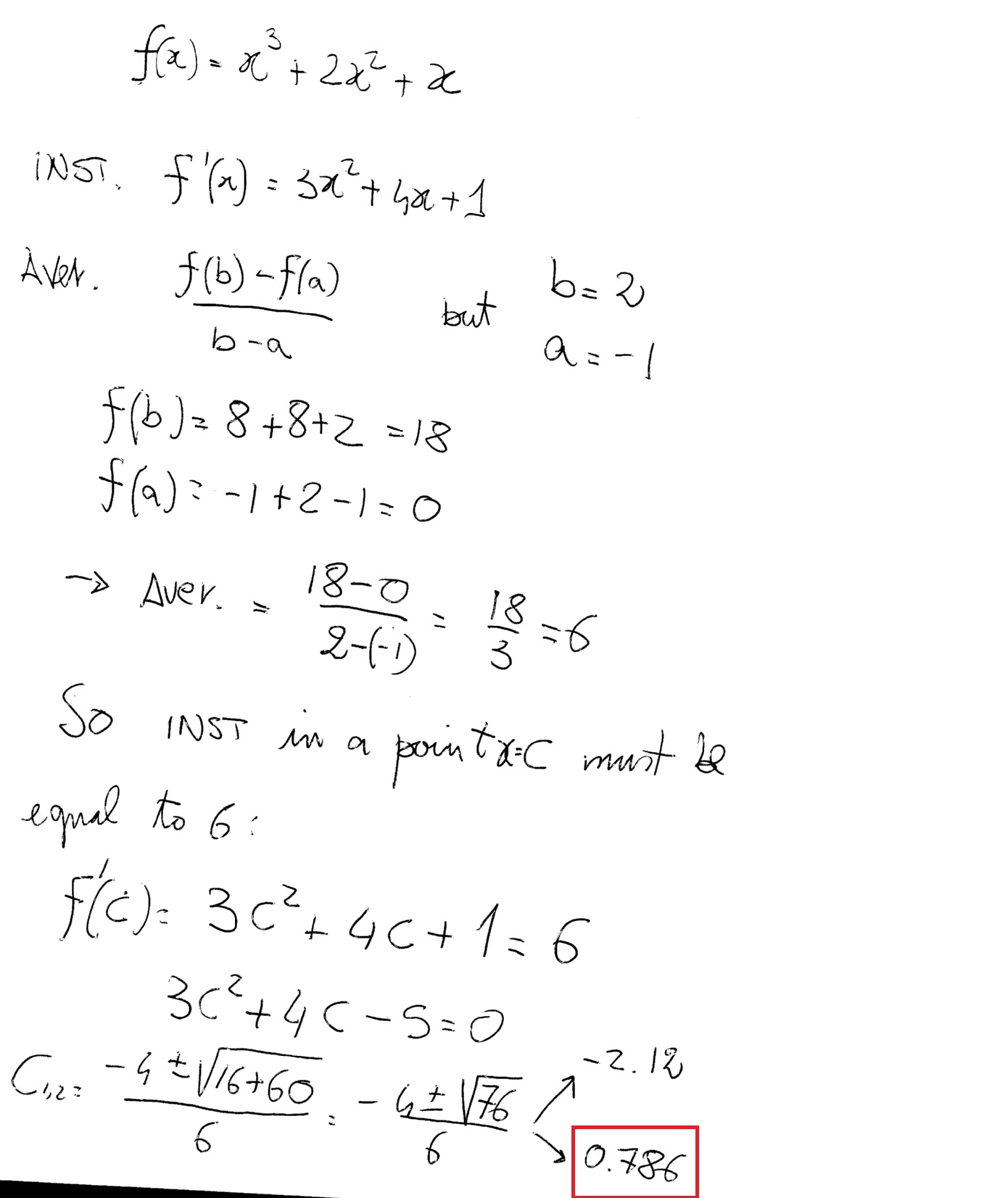
If F Is Defined By F X X 3 2x 2 X How Do You Find The Value Of X When The Average Rate Of Change Of F On The Interval X 1 To X

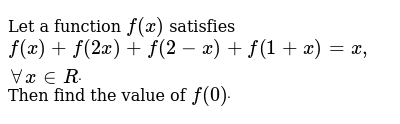
Let A Function F X Satisfies F X F 2x F 2 X F 1 X X x In Rdot Then Find The Value Of F 0 Dot

If F X X 1 X 1 Find F 2x In Terms Of F X Youtube

If F X 2x 2 3x 5 Then What Is F 0 3f 1 Equal To Youtube

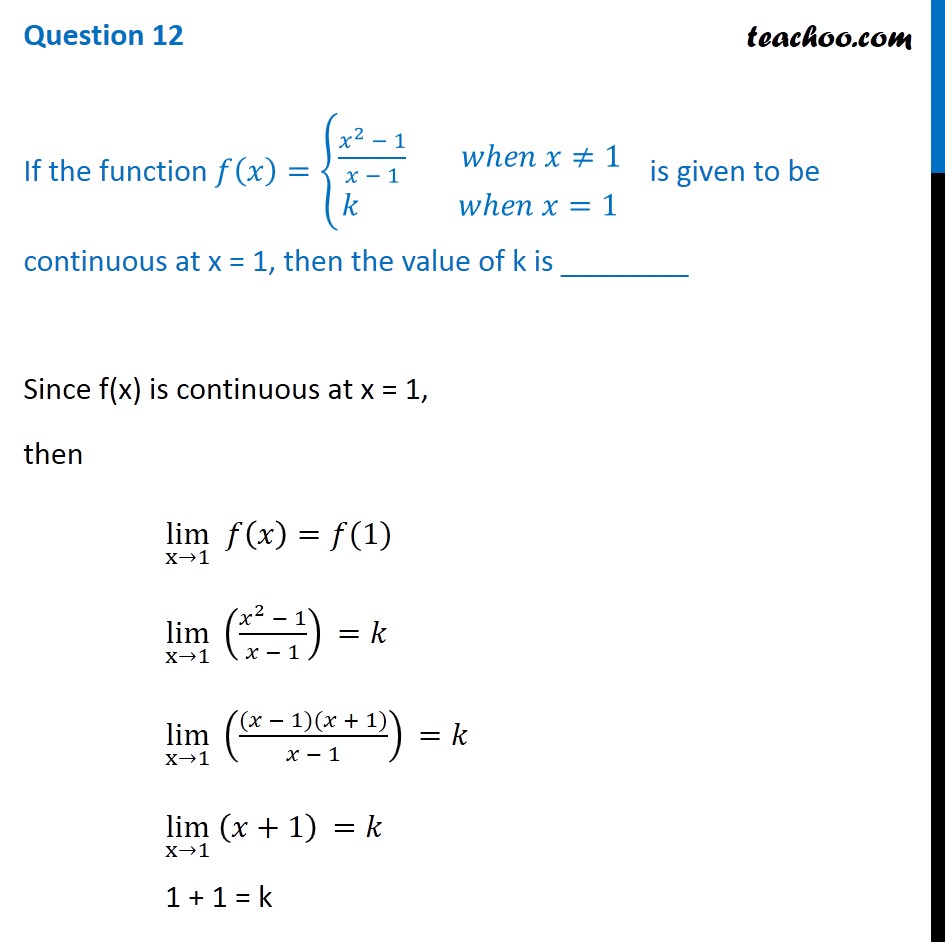
If The Function F X X 2 1 X 1 When X 1 K When X 1

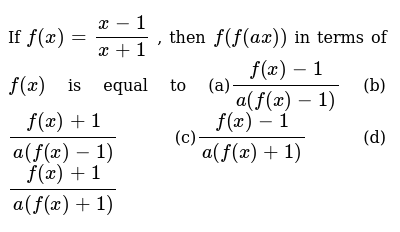
F X X A X A X A1 X A X Ax Ax A At X A Iv F X X A

If F X X 1x 1 Then F 2x In Terms Of F X Is

If F X A X 1 X A 1 2x Then The Value Of F 0 Is Brainly In

Solved If F X Equal To X Power 4 4 X Cube 3x Square 2x 1 Then Find Whether Fox F 1 F 2 Brainly In

14 If 2x 3x 1 Then The Values Of X Are Equal To Chegg Com

If F X X 2x 2 Evaluate F 2 F 1 F 1 2 Brainly In

What Is The Set Of All Points Where The Function F X X 1 X Is Differentiable Quora

Www Ebnet Org Cms Lib Nj Centricity Domain 816 Answer key ii Pdf

Find The Value Of A If The Function F X Defined By F X 2x 1 X 2 And A X 2 And X 1 X 2 Is

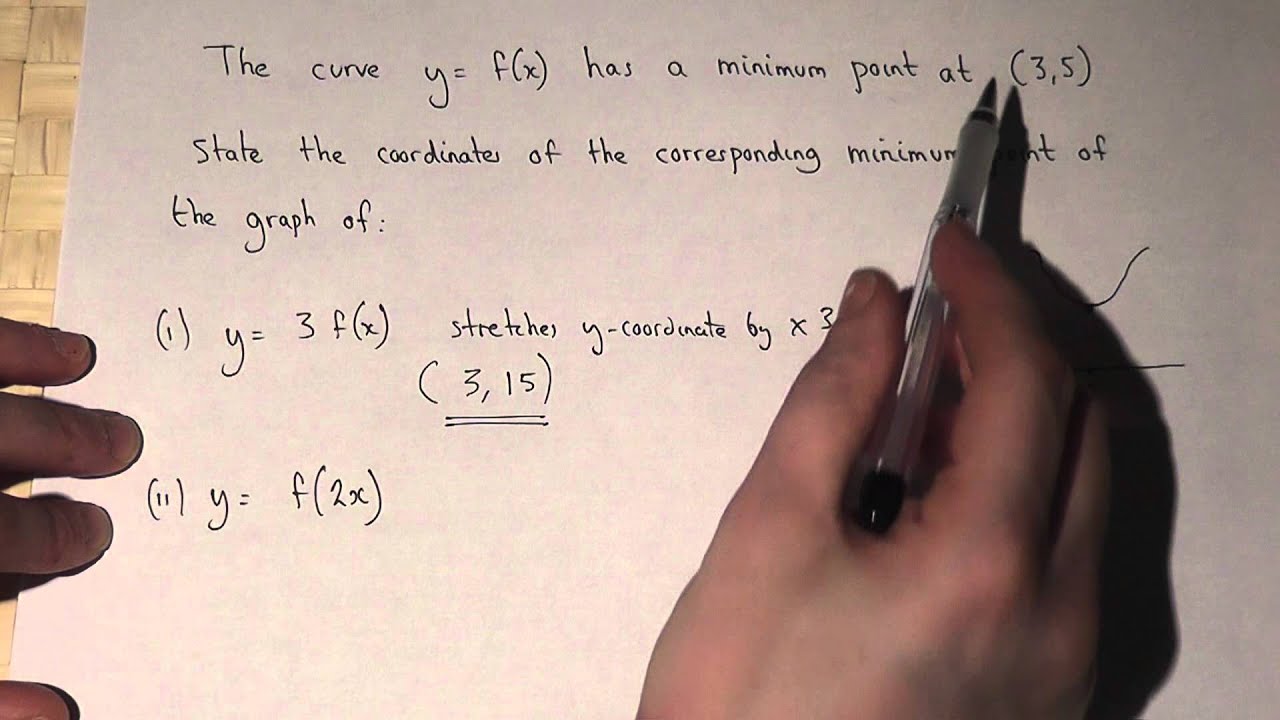
Graph Transformations Y 3f X And Y F 2x Youtube

If F X X 1 X 1 Then F 2x Is Equal To Maths Relations And Functions Meritnation Com

If F X 1 2x 3 Find F 3 Youtube

What Is The Range Of F X X 1 X 1 Quora

If F R R Is Defined By F X 2x 3 How Do You Prove That F Is A Bijection And Find Its Inverse Quora

How Do You Determine Whether The Function F X Ln X 2 7 Is Concave Up Or Concave Down And Its Intervals Socratic

What Is The Value Of F P 2 If F X 1 Cosx 1 Cosx Quora

If F X F 1 X F X F 1 X X Belongs To R 0 Where F X Is A Polynomial Function And F 5 126 Then What Does F 3 Equal Quora

If F X 3 X 1 X 2 3x Find F 0

Www Portnet Org Cms Lib6 Ny Centricity Domain 276 1st and 2nd derivative test 1 Pdf

If F 0 1 F 2 3 F 2 5 And F 0 Is Finite Then Int 0 1 Xf 2x Dx Is Equal To Youtube

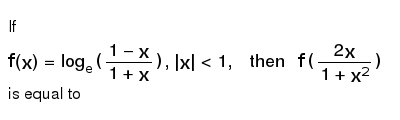
If F X Log E 1 X 1 X X Lt 1 Then F 2x 1 X 2

Example 16 Let F X X2 And G X 2x 1 Find F G Fg F G

If F X X 1 X 1 Then F 2x Is Equal To Youtube

If F 3x 4 5x 8 What Is The Inverse Of X I E F 1 X Quora

Find Gof And Fog If F X 8x 3 And G X X 1 3 Brainly In

If F X 2x And G X X 2 2 1 Then Which Of The Following Can Be Discontinuous Function

If The Function F X X 2 1 X 1 When X Ne1 K When X 1 Is Given To Be Continuous At X 1 Then The Value Of K Is

If F X X 1 X 1 X Is Not Equal To 1 Then Show That F F X 1 X Where X Is Not Equal To 0 Brainly In

If F X 3 X 2 And G X 1 X Then F Chegg Com

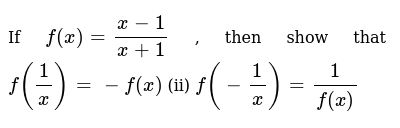
If F X X 1 X 1 Then Show That F 1 X F X Ii F 1 X 1 F X

A Function F Is Even If F X F X For All X In The Chegg Com

If F X 2x 3 G X X 3 5 Then Find Fog And Show That Fog Is

If F X X 3x 1 Find Xer Such That F 2x F X Brainly In

If F X X 1 X And F 1 5 2 Then F X Brainly In

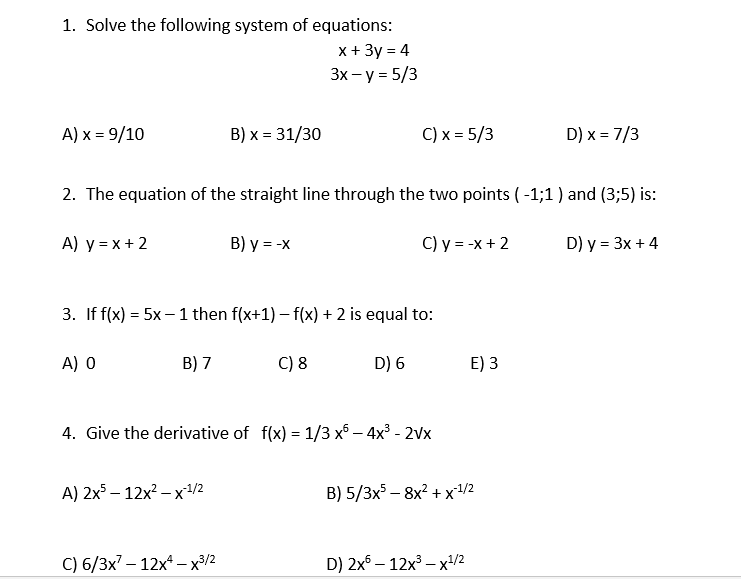
1 Solve The Following System Of Equations X 3y 4 Chegg Com

If F X 1 X 2 3x 2 Then What Is The Value Of F X Quora

Question 5 2 Pts If F X X 2 X 1 Then F X F X 1 Is Chegg Com

What Is The Inverse Of F X X 2 2x 2 Quora

Inverse Functions

If F X Log 1 X 1 X Then Show That F X F Y F X Y 1 Xy Brainly In

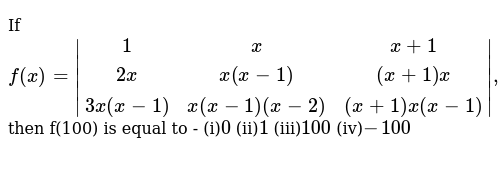
If F X 1 X X 1 2x X X 1 X 1 X 3x X 1 X X 1 X 2 X 1 X X 1 Then F 100 Is Equal To I 0 Ii 1 Iii 100 Iv 100

Is Function Of F X X 2 1 X 1 Continuous At X 1 Quora

Verifying Inverse Functions By Composition Not Inverse Video Khan Academy

If F X 1 X 2 3x 2 Then What Is The Value Of F X Quora

Ex 1 3 6 Show F X X X 2 Is One One Find Inverse Of F

If F X Log 1 X 1 X F 2x 1 X 2 2f X

D 1 E 30 1 Iff X X 2x 1 Then F 2 A Chegg Com

What Is The Inverse Of F X X 2 2x 2 Quora

If F X 2x 1 X Then Show That F Sin X 2 Sin X Its A Maths Question From Isc 11th Book Brainly In

If F X Log 1 X 1 X Show That F 2x 1 X 2 2f X Sarthaks Econnect Largest Online Education Community

24 If F X Ve 1 Then F 0 C 厄 1 D Ax 2 2e Chegg Com

If F X 1 1 X Find Fofof X

If F X X 1 X 1 Then Show That F 1 X F X Ii F 1 X 1 F X

If F X X 2 5x 1 Then Evaluate F 2 F 1 F 1 3 Brainly In

If F X Ax 2 Bx C F X 1 F X X 1 Is An Identity What Are The Values Of A B Quora

If F X 1 1 X Then F F F X Is Equal To

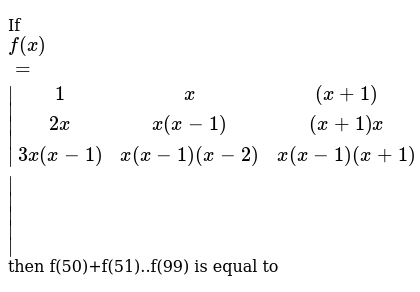
F X X X 1 2x 1 Then F X F X 1

If The Function F R R Defined By F X 4 X4 X 2 Then Show That F 1 X 1 F X And Hence Deduce The Value Of F 14 2f 12 F 34

Let F X X 1 X 1 Then F Is Differentiable In Youtube

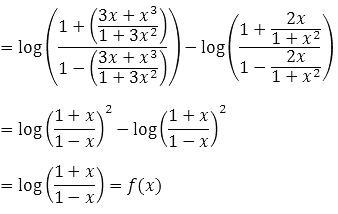
If F X Log 1 X 1 X Where 1 X 1 Then F 3x X 3 1 3x 2 F 2x 1 X 2 Is Equal To

If F X 1 X X 1 2x X X 1 X 1 X 3x X 1 X X 1 X 2

If G X Is The Inverse Function Of F X And F X 11 X 4 Then G X Is

If F X X 1 X 1 Then Prove That F 2x 3f X 1 F X 3 Brainly In

If F X 3x 10 Which Expression Represents F 2x 3 A 2x

Let The Function F Be Defined By F X 2x 1 1 3x Then F 1 X Is

If F X 1 X X 1 2x X X 1 X 1 X 3x X 1 X X 1 X 2 X 1 X X 1 Then F 100 Is Equal To Sarthaks Econnect Largest Online Education Community

If F X Then F 1 F 2 F 3 F 100 Is Equal To

If F X 2x 1 3x 2 Then F F 2 Is Equal Lto